Cycloidal tooth profile

What is cycloid?

The cycloid is defined as the locus of a point on the circumference of a circle that rolls without slipping on a fixed straight line.
In this type, the faces of the teeth are epicycloids and the flanks are the hypocycloids. 

Now one question arises in your mind that what is epicycloids and hypocycloids? Let we check 

What is epicycloid?
An epicycloid is the locus of a point on the circumference of a circle that rolls without slipping on the circumference of another circle. 

What is hypocycloid?
A hypocycloid is the locus of a point on the circumference of a circle that rolls without slipping inside the circumference of another circle. 

Formation of cycloidal tooth :

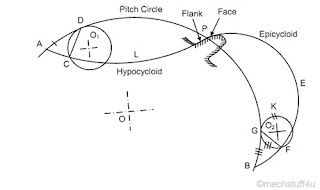
A circle O₁ rolls inside another circle APB is called pitch circle. At the start, the point of contact of the two circles is at A. As the circle O₁ rolls inside the pitch circle, the locus of the point A on the circle O₁ traces the path ALP which is called hypocycloid. A small portion of this curve near the pith circle is used for the flank of the tooth.  

The line joining the generating point A with the point of contact of the two circles is normal to the hypocycloid when the circle O₁ touches the pitch circle at D, the point A is at C and CD is normal to the hypocycloid ALP. 

In the same way, if the circle O₂ rolls outside the pitch circle, starting from P, an epicycloid PFB is obtained.

A small portion of the curve near the pitch circle is used for the face of the tooth also. 

Some key points :

• The path of approach is equal to the arc of approach. 
• The path of contact is equal to the arc of contact. 
• In case of cycloidal teeth, the pressure angle varies from the maximum at the beginning of the engagement to zero when the point of contact coincides with pitch point and then again increased to a maximum in the reverse direction. 
• Since cycloidal teeth are made up of the two curves, it is very difficult to produce accurate profiles. This has rendered this system obsolete.